 [RL] Feature Construction for Linear Methods
[RL] Feature Construction for Linear Methods
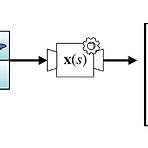
(해당 포스트는 Coursera의 Prediction and Control with Function Approximation의 강의 요약본입니다) - 관련된 책 내용 : 9.4 - Linear Methods : 9.5.3 - Coarse Coding : 9.5.4 - Tile Coding : 9.7 - Non-linear Function Approximation : ANN 이전 포스트에서 설명한 Linear Function Approximation은 일반적으로 표로 표현된 value function을 어느 유사한 function으로 근사함으로써 효율성을 가져오자는 취지에서 나온 것이고, 보통 이 function을 조절하는데 weight vector w를 사용한다. 그래서 이에 대한..
(해당 포스트는 Coursera의 Prediction and Control with Function Approximation의 강의 요약본입니다) 이전 포스트들을 통해서 설명하고자 했던 것은 기존의 Q-table과 같은 Tabular 방식이 아닌, Value를 하나의 Function, 즉 Value Function으로 근사하는 방법이 존재하고, 이때 이 근사된 Value Function과 실제 Value Function과의 오차를 줄일 수 있는 방법으로 Gradient Descent를 적용할 수 있다는 것이었다. 그래서 Function Approximation을 Monte Carlo에 적용한 Gradient MC과 제한적이기는 하나, Gradient를 TD Learning에 적용한 Semi-Gradien..
- Total
- Today
- Yesterday
- Windows Phone 7
- PowerPoint
- reward
- Kinect
- Distribution
- Kinect for windows
- Variance
- Gan
- 한빛미디어
- Offline RL
- 딥러닝
- SketchFlow
- processing
- bias
- Expression Blend 4
- End-To-End
- arduino
- dynamic programming
- RL
- Pipeline
- Policy Gradient
- Off-policy
- 파이썬
- Kinect SDK
- ai
- DepthStream
- ColorStream
- TensorFlow Lite
- 강화학습
- windows 8
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | ||||||
| 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| 9 | 10 | 11 | 12 | 13 | 14 | 15 |
| 16 | 17 | 18 | 19 | 20 | 21 | 22 |
| 23 | 24 | 25 | 26 | 27 | 28 | 29 |
| 30 | 31 |
